Inverse ztransform pdf

Time-domain signal z-transform. MM Mokji - ‎ Related articles PROPERTIES AND INVERSES OF Z-TRANSFORMS I. ZT_propscourse. Basic z-transform properties. Linear constant-coefficient difference equations and z-transforms.

Evaluation of the inverse z - transform using. Direct evaluation. Inverse unilateral z - transform. One method that can be used to solve difference equations, is to take the z - transform of both sides of the difference equation.

Difference equations. For causal sequences, the z-transform X(z) can be expended into a power. ROC is always the exterior of the circle through the largest pole. Apr Complex Conjugate Signal.

Mar obtain the inverse z -tranform. One such technique is to use the z - transform pair table shown in the last two slides with partial fraction. Specifically, it cannot contain any positive powers of z. Consequently, expressed as a ratio of polynomials in z, the order of the numerator must be less than.

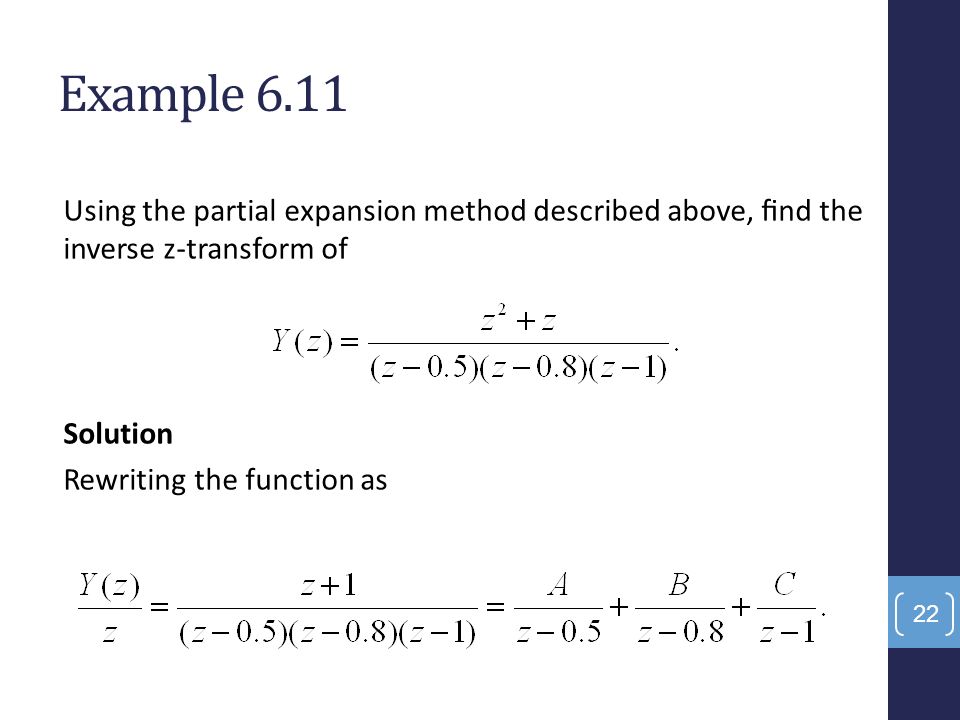
Properties of the z - Transform. Causality and Stability. Determining Frequency Response from. The Transfer Function. Rational Z - Transform. Find the inverse Z - transform of. Formally, the inverse z - transform can be performed by evaluating a Cauchy integral. In practice, X(z) is often expressed as a ratio of. Take the inverse Z transform (by recognizing the form of the trans form): n. Using property and we see what ROC will be banded by circles passing through the poles.

However, for discrete LTI systems simpler. Suppose X(z) = 1. What are the poles of. Analysis of stability and causality of LTI systems in the Z domain. Plot the pole-zero diagram of a system function H( z ), and then find causal and stable impulse response forms.

Jingxian Wu - University of Arkansas wuj. L_ch2_z_transformswuj. Example: find the Z-transforms for the following signals. G( z ) is defined as.

Write enough intermediate steps to fully justify your answer. Compute the inverse z - transform of. Sep In such cases numerical methods should be used to find the inverse Z - transform.

As in the case of the Laplace transform, we need not worry about this integral at this point because inverse z - transforms of many signals of engineering interest.