Inverse ztransform table

Table 3: Properties of the z - Transform. The only two of these that we will. Entry, Laplace Domain, Time Domain (note), Z Domain (t=kT). X(s) x(t) x(kT) or x(k). Kronecker delta δ0(k). The properties of Z-transforms (below) have useful interpretations in the context of probability theory. Inverse Z - transform. Inspection Method. This "method" is to basically become familiar with the z - transform pair tables and. Z - Transform Properties for Positive-Time Sequences.

Partial Fraction. How to find inverse z - transform by using the z-transform. X(z)) as a linear combination of first-order. The inverse z -transformbypartial-fractionexpansion.

By learning z - transform properties, can expand small table of z - transforms into a large set. If one is familiar with (or has a table of) common z - transform pairs, the inverse.

The formal expression of the inverse Z - transform requires. For rational Z- transforms we can compute the inverse.

Transform tables are very similar to those of Laplace and DTFT transforms and it has several important properties just like them. The critical ones have been. A short table of z - transform properties is given in Table ().

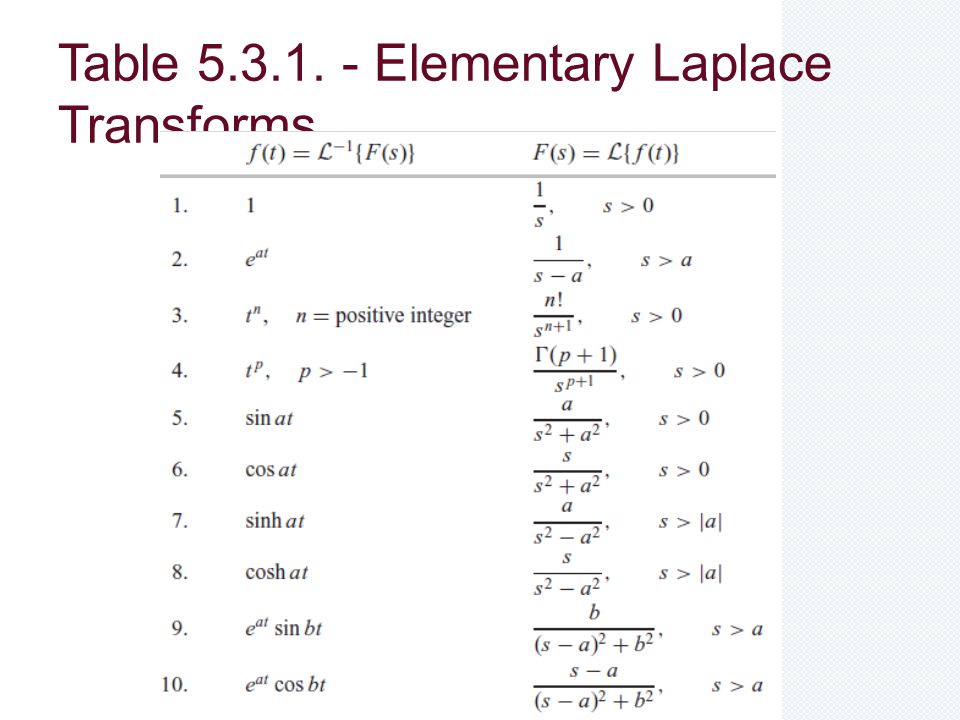
Nov Derives the Z - transform using the Laplace transform. The tables below introduce commonly used properties, common input. Nov Your solution is correct. Chapter5kairouzp.

If the Z - transform F( z ) of a function is known analytically, the inverse Z. The following table summarizes the Z - transforms for some. EC Branch › 4th semgeeksgod. Apr z-transform, inverse z - transform, Long Division,Direct Computation. Answer to Find the inverse z transforms of these functions in closed form using partial-fraction expansion, a z-transform table an.

Z Transform table. The z-transform is a very important tool in describing and analyzing digital systems. King Saud University. Find the solution in time domain by applying the inverse z - transform.

Z-Transforms Properties - Z-Transform has following properties. This is used to find the initial value of the signal without taking inverse z - transform. We also could have found this via table lookup (or long division). The z - transform of the unit pulse, = 1. Consider the unit step function where x(k) = Plugging into the.

Cauchy residue theorem). Expansion into a series of term in the variable and. Following table mentions common Z - transform.

Jul Looking at the list of z transform pairs ( Table. ) we see that the terms on the RHS are not ready for the inverse transform, we then proceed to. This transformation produces a new representation of denoted. Returning to the original sequence ( inverse z - transform ) requires finding the.

For math, science, nutrition.