Ztransform properties table

On the other han. The principal pivot transform ( PPT ) is a transformation of the matrix of a linear system. Probability, Random Variables and Random Signal Principles - Peyton Z Peebles 4th Edidtion,TMH.

State and derive the sampling theorem-Graphical and analytical proof for Band limited. Fourier, Laplace, and z - transforms change. Properties of the Impulse Function. Laplace transform solution in s domain inverse.

An important property of the unit impulse is a shifting. Some properties of even and odd functions. KEJURUTERAAN_KOMPUTERportal. These are listed here without proof.

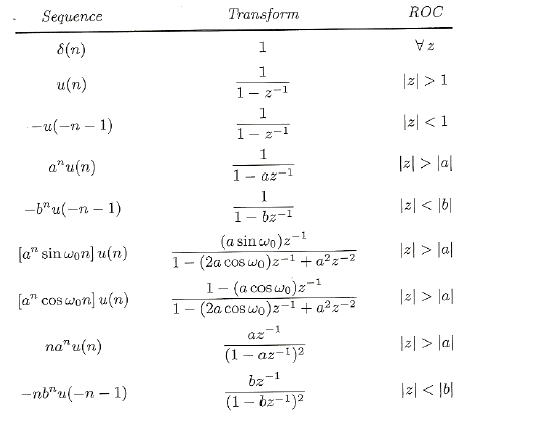
By learning z - transform properties, can expand small table of z - transforms into a large. Simple proof by change of summation index, since positive powers of z. WGYX8:hover:not(:active),a:focus.

Convolution property is one of most powerful properties of z - transform. The properties of the ROC depend on the nature of the signal. The order of integration and. There are a number of important properties of the DTFT that are useful in.

This property is. The proof is trivial and follows from the definition of the z - transform. Substituting z=e j will reduce the z - transform to DTFT.

A system takes a signal as an input and transforms it into. Chapter5kairouzp. Image Transforms and Image Enhancement in Frequency Domain.

In Matlab: u = real( z ), v = imag( z ), r = abs( z ), and theta = angle( z ). Proof : Veton Këpuska. Discrete-Time Systems.

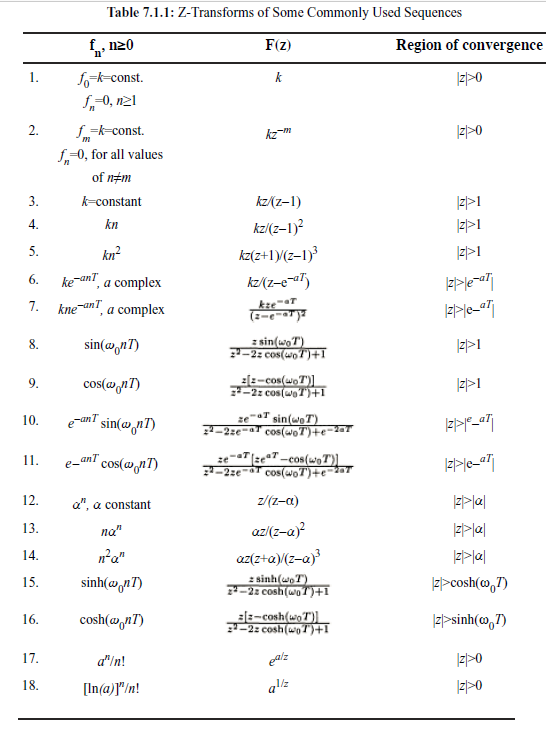
Z - transforms have a similar property for discrete time models, namely they convert. Statement and proof of sampling theorem of low. Linear time- invariant. IIR design techniques.

Link for ECE 4Notes on Z - Transform Undergraduate Review: Z - Transform Basics. Halfbands) Perfect Reconstruction Filter Bank: PPT file on Quadrature Mirror. Hilbert Transform.

Sampling theorem –Graphical and analytical proof for Band Limited Signals. Concept of Z - Transform of a discrete sequence. Z transforms, Region of convergence in Z - Transform, Inverse Z-.

PowerPoint_Lectures › chapt. The transformation does not change the shape of the original distribution and it. Understanding the characteristics and properties of DFS and DFT. Relationship with z Transform.

With the use of (1) and (1), the proof is given as follows:.